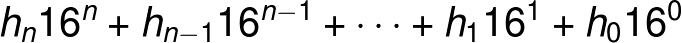Hexadecimal
Converting To/From Decimal
By now, we know how to convert about 16-or-so values between decimal and hexadecimal. To convert bigger numbers, here are some tricks we use.
Converting Decimal to Hex
Converting from decimal to hex involves a lot of division and remainders. If you've pushed long division out of your brain, wiki's always there to help you brush up.
The steps to convert a number, let's call it N, from decimal to hex look something like this:
- Divide N by 16. The remainder of that division is the first (least-significant/right-most) digit of your hex number. Take the quotient (the result of the division) to the next step.
- Note: if the remainder is 10, 11, 12, 13, 14, or 15, then that becomes the hex digit A, B, C, D, E, or F.
- Divide the quotient from the last step by 16 again. The remainder of this division is the second digit of your hex value (second-from-the-right). Take the quotient from this division to the next step.
- Divide the quotient from step 2 by 16 again. The remainder of this division is the third digit of your hex conversion. Noticing a pattern?
- Keep dividing your quotient from the last step by 16, and storing the remainder until the result of a division is 0. The remainder of that division is your hex value's left-most, most-significant digit.
Decimal-to-Hex Example: Convert 61453
Enough math-speak, let's work an example. Let's convert 6145310 to hexadecimal:
- Divide 61453 by 16. The result is a quotient of 3840, and a remainder of 13. That remainder becomes our first, right-most, least-significant hex digit -- D. Take 3840 to the next step.

- Now divide 3840 by 16. The resulting quotient is 240 with a remainder of 0. Our second hex digit is 0, and we take 240 to the next digit.

- Divide 240 by 16, and you'll get 15 with another 0 remainder. Our third hex digit is 0, and take 15 to step 4.
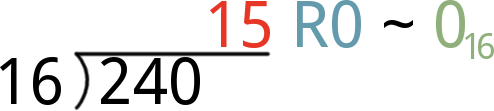
- Finally, divide 15 by 16. That'll produce the 0 quotient we've been waiting for, with a remainder of 15. That remainder means the hex digit for this position if F.
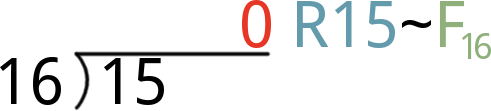
Finally, combine all four hex digits to create our hex value: 0xF00D.
Converting Hex to Decimal
There's an ugly equation that rules over hex-to-decimal conversion:
There are a few important elements to this equation. Each of the h factors (hn, hn-1) is a single digit of the hex value. If our hex value is 0xF00D, for example, h0 is D, h1 and h2 are 0, and h3 is F.
Powers of 16 are a critical part of hexadecimal. More-signficant digits (those towards the left side of the number) are multiplied by larger powers of 16. The least-significant digit, h0, is multiplied by 160 (1). If a hex value is four digits long, the most-significant digit is multiplied by 163, or 4096.
To convert a hexadecimal number to decimal, you need to plug in values for each of the h factors in the equation above. Then multiply each digit by its respective power of 16, and add each product up. Our step-by-step approach is:
- Start with the right-most digit of your hex value. Multiply it by 160, that is: multiply by 1. In other words, leave it be, but keep that value off to the side.
- Remember to convert alphabetic hex values (A, B, C, D, E, and F) to their decimal equivalent (10, 11, 12, 13, 14, and 15).
- Move one digit to the left. Multiply that digit by 161 (i.e. multipy by 16). Remember that product, and keep it to the side.
- Move another digit left. Multiply that digit by 162 (256) and store that product.
- Continue multiplying each incremental digit of the hex value by increasing powers of 16 (4096, 65536, 1048576, ...), and remember each product.
- Once you've multiplied each digit of the hex value by the proper power of 16, add them all up. That sum is the decimal equivalent of your hex value.
Hex-to-Decimal Example: Convert 0xC0DE
Here's an example of a four-digit hexadecimal value, 0xC0DE, that we want to convert to decimal. Creating a table and sorting the digits into separate columns can make the conversion process easier:
| Hexadecimal Digit | Notes | ||||
|---|---|---|---|---|---|
| Digit Positions (n) | 3 | 2 | 1 | 0 | These values are statically assigned, they grow to the left. |
| Hex Digits Sorted | C | 0 | D | E | This part's easy, plug your hex values in from right-to-left. |
| Convert A-F | 12 | 0 | 13 | 14 | Convert hex values A-F to 10-15. |
| Multiply by 16n | 12 × 163 | 0 × 162 | 13 × 161 | 14 × 160 | The exponent of 16 is the position, n. |
| Resulting Products | 49152 | 0 | 208 | 14 | The product of hex digit and the power of 16. |
| Sum Up All Products | 49374 | Our decimal equivalent! | |||
There you have it. CODE16 = 4937410!
This table method is perfect for keeping all of your hex digits, positions, and powers-of-16 in line. To convert larger hex numbers, just add a columns to the left and increase n.
Now that you know how to do it by hand, save yourself a little time and use a calculator.